Jan 8 2018
In literature, the prospective presence of extra dimensions was debated in “Flatland: A Romance of Many Dimensions” (1884), Edwin Abbott’s satirical novel depicting the Victorian society in 19th-century England as a hierarchical two-dimensional realm, lacking the ability to realize its narrow-mindedness owing to its lower dimensional characteristic.
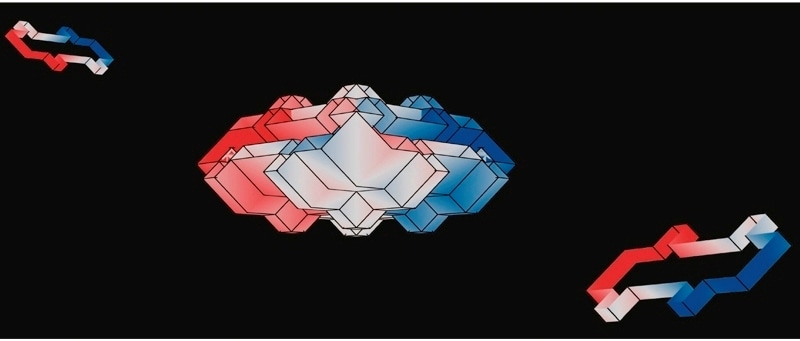 Illustration of a hypothetical device for studying the quantum Hall effect in 4D systems. Two 2D Hall bars (left/right) are combined in orthogonal subspaces to form a 4D quantum Hall system (center). This 4D sample is depicted by encoding the fourth dimension in the color of a surface in three spatial dimensions with red depicting positive values and blue negative ones. (Photo credit: LMU/MPQ)
Illustration of a hypothetical device for studying the quantum Hall effect in 4D systems. Two 2D Hall bars (left/right) are combined in orthogonal subspaces to form a 4D quantum Hall system (center). This 4D sample is depicted by encoding the fourth dimension in the color of a surface in three spatial dimensions with red depicting positive values and blue negative ones. (Photo credit: LMU/MPQ)
In contrast, in the field of physics, the probability of the existence of greater than three spatial dimensions in our universe was first put forward based on Albert Einstein’s theory of general relativity in the 1920s. Modern string theory, which attempts to integrate Einstein’s concepts with quantum mechanical laws, even hypothesizes the existence of nearly 10 dimensions. In an entirely distinct context, a team of international scientists headed by Professor Immanuel Bloch (LMU/MPQ) and Professor Oded Zilberberg (ETH Zürich) has at present exhibited a method to observe physical phenomena hypothesized to be present in higher dimensional systems in equivalent real-world experiments. The team used ultracold atoms confined inside a periodically modulated two-dimensional superlattice potential to observe a dynamical version of an innovative kind of quantum Hall effect that is proposed to exist in four-dimensional systems. The study was published on January 4, 2018 in the Nature journal.
The Hall effect takes place upon moving charged particles in a two-dimensional plane under the effect of a magnetic field. A Lorentz force generated by the magnetic field deflects the particles in a direction orthogonal to their movement. This results in the occurrence of a transverse Hall voltage. In the year 1980, Klaus von Klitzing astonishingly found out that at lower temperatures and highly strong magnetic fields, this voltage could take only specific quantized values. In addition, the values are similar regardless of the individual characteristics of the experimental sample. This remarkable fact was in the meantime demonstrated to be due to the topology of the quantum mechanical wave functions outlining the functions of electrons at very low energies. In 2016, David Thouless won the Nobel prize in physics for this seminal work.
An essential precondition for the quantum Hall effect was found to be the sample’s two-dimensional geometry. One can demonstrate that normally this phenomenon cannot occur in three-dimensional systems, as represented by the reality that the direction transverse to the velocity of the particles is not distinctively defined in three dimensions. Hence it was considered that this effect is imminent in two dimensions. However, two decades after the first discovery, theoretical physicists hypothesized that an identical effect can also occur in four-dimensional systems; in this case, more astonishing characteristics, including an innovative non-linear Hall current, were proposed. For many years, this idea was largely thought to be just a mathematical curiosity, which could not be accomplished through real-time experiments, in spite of its far-reaching inferences. For instance, Weyl semimetals as well as topological insulators, the most significant discoveries in condensed matter physics in the past few years, can be made by using 4D quantum Hall models.
In the year 2013, Oded Zilberberg and his colleagues found that important expressions of the 4D quantum Hall effect must be also visible in distinctive time-dependent systems in two dimensions, the well-known topological charge pumps, which form a dynamical category of the higher dimensional model. This knowledge generalized a concept, which was also put forward by David Thouless. In the year 1983, Thouless demonstrated that a quantized transfer of particles can be produced by periodically regulating a one-dimensional system and that this reaction is mathematically analogous to the two-dimensional quantum Hall effect. As a result, when two such systems are integrated in orthogonal directions, it must be feasible to observe the non-linear Hall current proposed in 4D.
At present, Immanuel Bloch’s team has accomplished this. Initially, an atomic cloud is cooled down nearly to absolute zero and positioned inside a 2D optical lattice. This optical lattice is developed by interference between retro-reflected laser beams of a specific wavelength along two orthogonal directions. The ensuing potential is similar to an egg-carton-like “crystal of light” wherein the atoms are free to move. A superlattice is formed by adding another laser beam with a distinct wavelength in every direction. The team was able to execute the predicted 2D topological charge pump by forming a very small, constant angle between the different wavelength beams along one axis while simultaneously, altering the shape of the potential in a dynamic manner in the orthogonal direction by moderately modifying the additional laser beam’s wavelength.
When the potential is regulated in time, the atoms principally travel in the regulated direction in a quantized manner—the linear, or one-dimensional, response related to the two-dimensional quantum Hall effect proposed by Thouless. However, apart from this, the Munich researchers also noticed a minute drift in the transverse direction, although the lattice potential in this direction stays static all through the experiment. This transverse motion is analogous to the non-linear Hall response—the crucial aspect of the 4D Hall effect. Through cautious investigation and monitoring of the positions of the superlattice in which the atoms are positioned during the process, the researchers could further show that this motion is quantized, thus exhibiting the quantum characteristic of the Hall effect in 4D.
At present, the outcomes have been reported in the Nature journal together with corresponding research by an American research group, which adopted photonic structures to investigate the complex boundary phenomena that go along with this motion due to the 4D quantum Hall effect. Jointly, both the studies offer the first ever experimental view into the physical aspects of higher dimensional quantum Hall systems, which provide various astonishing future probabilities, which include basic questions on our knowledge of the universe, such as the generation of cosmic magnetic fields, the interplay of quantum correlations and dimensionality, and quantum gravity, for which 4D quantum Hall systems have been suggested as toy models.