AZoQuantum talks to Dr. Melvin Vopson about his research around the quantification of Information within the known universe.
Could you please tell us a little about your background and how you came to be involved with this research?
Beginning with my doctoral work and continuing after obtaining my Ph.D., my research background has mostly revolved around various topics in condensed matter physics with some emphasis on digital data storage technologies, ferroic and multiferroic materials, but also studies of conduction properties, optical properties of solids, development of novel metrologies and thin-film nano-technologies.
These diverse studies implied experimental, applied, and some theoretical research work. Some of my most important contributions are the theoretical discovery of the Multicaloric Effect, the development of new polarization reversal model in polar dielectrics, and various studies of multiferroics materials.
Recently, I became interested in the Physics of Information, while I was working on a different project involving possible multi-state digital data storage technologies based on anti-ferroelectric solids. While working on the multi-state memory device concept, my main issue was how to allocate bit memory states to a memory cell that has more than 2 coexisting physical states (all digital memory technologies today have memory cells with two possible states per cell, 1 and 0, and one bit per cell).
This is when I introduced myself to Shannon’s Information Theory and Landauer’s principle. Stimulated by these fascinating theories, I managed to connect some dots and I made my own contributions to this interesting field of research.
Can you please describe the connection between the physical universe and Information?
This is a very complex question. The connection between the physical Universe and Information is profound, because the Information has been proven to be physical. Since Information is physical, it must be an integral part of a physical Universe.
Things get a bit more complicated when one starts to contemplate what Information is and what its role is in the Universe. It appears that Information is the “blueprint” of everything. When talking about biological matter, Information’s role is undeniable, and it manifests as the storage of biological data in the RNA / DNA that makes up any form of life of any size.
What about non-organic matter? Let’s examine an electron. It has the same mass, charge and spin regardless of whether it is measured on Earth, at the edge of our galaxy or anywhere else in the Universe. So it begs the question: How does an electron on Earth come to be identical to an electron in a different galaxy?
Then we have the laws of Physics. These are equally applicable and identical anywhere in the Universe.
One possible explanation is that there is an Information Field that permeates the whole Universe giving the blueprint of everything and anything in it. This is best articulated by Wheeler’s suggestion that: “…the Universe emanates from the information inherent within it…” or, “It from bit”.
On this principle, pure quantum states could be regarded as information, and this could be further extrapolated to something similar to a “matter DNA”, where every elementary particle making up everything in the Universe is labeled with its own defining characteristics. Shannon gave us a tool to estimate the value of this information, and the unit of information is the “bit”.
When looking at Physics and the Universe from this informational angle, and considering the mass – energy – information equivalence principle [https://doi.org/10.1063/1.5123794], which I formulated in 2019, stating that information is not only energy but it can have mass, then it appears plausible that Information could be in fact the dominant form of matter in the Universe.
I previously called Information the 5th state of matter alongside solid, liquid, gas and plasma, and I postulated that the missing dark matter could be in fact Information.
These views of the Universe are not new. In my recent article, I gave a brief introduction to other estimates of the Information content of the Universe, including Lloyd’s ideas suggesting that the whole Universe behaves as a giant computer
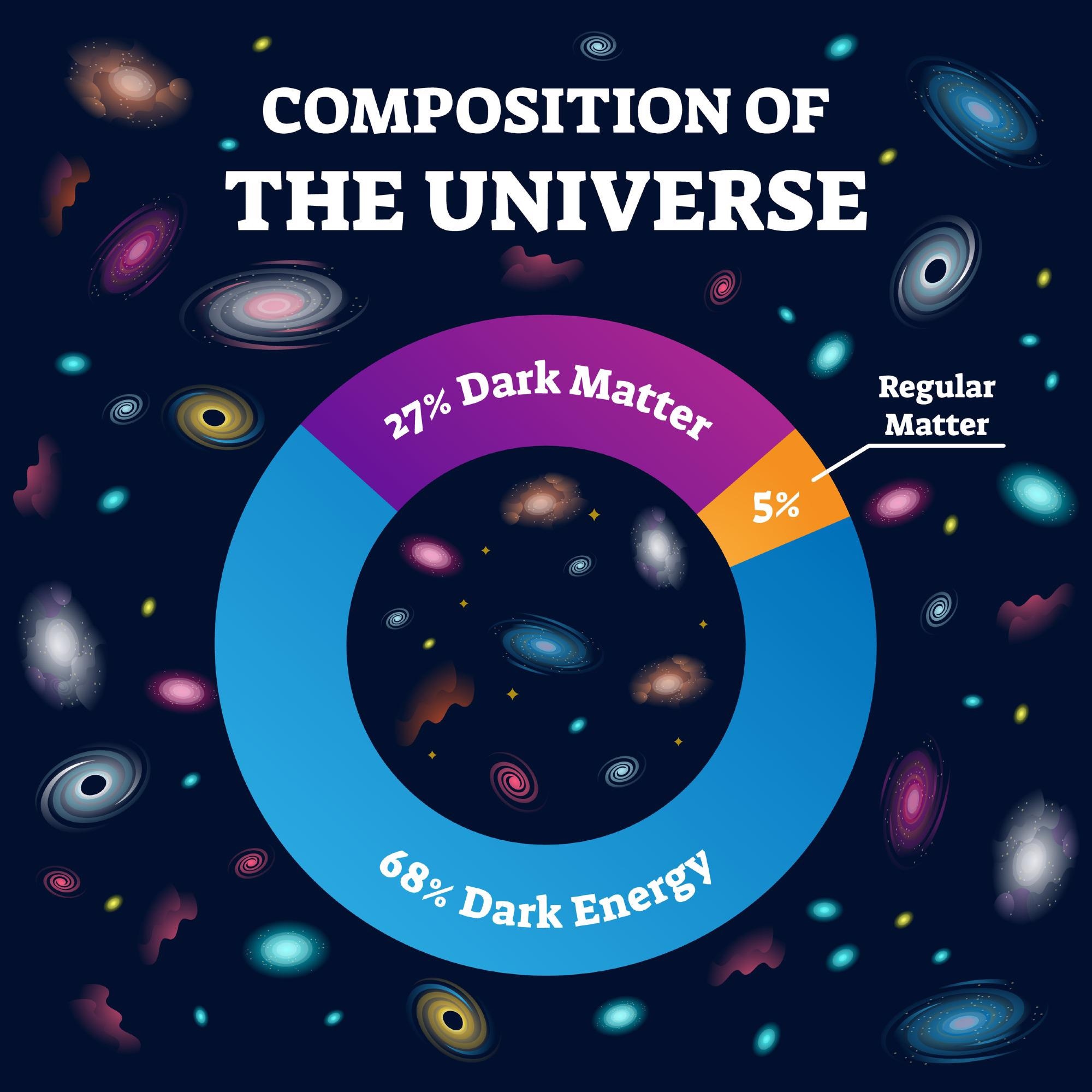
Image Credit: VectorMine/Shutterstock.com
What is meant by ‘Information’ in this context, and what is its significance?
In my studies, the concept of Information is strictly limited to the Shannon’s formalism. Under this formalism, any physical system, any set of events, anything that could be described by statistical probabilities, can have an information content, or can register information. We need to make a clear distinction between Information and “bits”.
Information is a physical property and the bits are the units of Information, i.e. Information and bits, distance and meters, temperature and Kelvin degrees, etc. The significance of this is huge, because if Information is physical, then we need to consider or reconsider the informational component of everything in Physics.
Why has it been difficult in the past to quantify the amount of Information contained in visible matter?
There have been a lot of different attempts to quantify the information content of the Universe, but they all took a rather different approach to mine and to each other. In my recent article, I gave a brief review of these estimates, most notable being those reported by Davies [Ref. 28], Wheeler [Ref. 8], Lloyd [Ref. 6] and Gough [Ref. 30], where references are in: https://doi.org/10.1063/5.0064475.
What did your process and formula look like that led you to be able to postulate a near-exact number?
We need to be careful here when talking about exact numbers. There is a reason nothing in my article has any certainties stated. The main issue with these estimates is that they are based on other estimates, including probabilities, and there is no way of having the exact values of parameters that go into the calculations.
What we have is our best estimates and the important things are the trends and the bulk estimates that could be used to guide future experimental testing, for example. Another issue that needs to be highlighted is the fact that uncertainties applied to cosmic scales, i.e. very large numbers, become almost meaningless.
For example, if one measures the temperature of a physical system at room temperature and gives an uncertainly of the measurement of 0.01% (or 1 in 10000), then any rational person would conclude that this measurement is extremely precise down to fractions of mK. However, if the same uncertainty were applied to a parameter described by a very large number, as we could consider the radius of the observable universe (44 × 1025 m), then the uncertainty would be ~ 1021 m, which is an enormous number.
You state that there are approximately 6 times 10 to the power of 80 Informational bits in the universe. Is this more or less you had expected?
This number is significantly less than I expected. My prime objective was to find the missing dark matter. According to one of my previous estimates, we need ~1093 bits of information to make up all the missing dark matter [https://doi.org/10.1063/1.5123794]. The number I came up with in my latest article is smaller than I expected.
Where is the missing stuff? It could be that there are things not accounted for in the estimate. It could be that information is stored in other forms/media, etc. It could be that the previous estimate was wrong. The hope is that this will stimulate some more research in the community and we will eventually find the answers soon.
Is it possible that this number is incorrect? What further experimentation would be necessary to provide a more definitive answer?
It is very possible. However, the exact value of information content per particle is not important. What is important is the overall range, the indicative value of information content that one would expect to find in a particle assuming the best compression mechanism as dictated by Shannon’s theory. Whether the exact value is 1.509 bits as reported in my article, 1.7 bits or 2.2 bits, it makes not much difference. Having an estimate allows one to start designing the experiments to check these ideas.

Image Credit: Marcel Drechsler/Shutterstock.com
Once the experiments are performed, they will either prove or disprove these ideas. Assuming the experiment yields a positive outcome, but the values are a bit off relative to the theoretical estimate, then we have grounds to go back and refine the theory further. I am now working on my next article in which I am proposing a novel experiment to test these predictions. Unfortunately I cannot disclose at this stage the experimental strategy, but this will be in the public domain very soon.
How do antiparticles and neutrinos, for example, affect this quantification if they are also considered?
I did not consider in my estimates anything unstable (particles of a very short lifetime, antiparticles, etc) or particles that only exist when artificially created, because such particles cannot be considered as participants to the observable Universe.
Hence, under my postulate, they cannot store information. Neutrinos and force carrier bosons have not been included because they do not have a non-zero rest mass. It has been postulated that these particles can transfer information, but they do not store information.
This is due to the mass – energy – information (M/E/I) equivalence principle [https://doi.org/10.1063/1.5123794] that requires information to have mass while it is stored in a medium. Hence, a particle with zero rest mass cannot store information. This is my view on this issue, but I read some recent studies citing my M/E/I principle, in which some researchers do consider photons as medium of information storage.
What is personally the most exciting implication of this new formula for you?
If you are referring to the formula that reproduces the Eddington number, then I am very excited about the fact that the formula can give the number of electrons, protons or neutrons in the Universe, while the Eddington number is an estimate of the number of protons only. The formula also allows for further refinements, if in future we get better estimates of some of the parameters incorporated in it.
Further Work By Dr. Vopson
Vopson, M. M. (2012). The multicaloric effect in multiferroic materials. Solid State Communications, 152(23), 2067–2070. https://doi.org/10.1016/j.ssc.2012.08.016
Vopsaroiu, M., Blackburn, J., Cain, M. G., & Weaver, P. M. (2010). Thermally activated switching kinetics in second-order phase transition ferroelectrics. Physical Review B, 82(2). https://doi.org/10.1103/PhysRevB.82.024109
Vopson, M. M. (2015). Fundamentals of Multiferroic Materials and Their Possible Applications. Critical Reviews in Solid State and Materials Sciences, 40(4), 223–250. https://doi.org/10.1080/10408436.2014.992584
Vopson, M. and Tan, X., 2016. Four-State Anti-Ferroelectric Random Access Memory. IEEE Electron Device Letters, 37(12), pp.1551-1554. https://doi.org/10.1109/LED.2016.2614841
Vopson, M., Caruntu, G. and Tan, X., 2017. Polarization reversal and memory effect in anti-ferroelectric materials. Scripta Materialia, 128, pp.61-64. https://doi.org/10.1016/j.scriptamat.2016.10.004
About Dr. Vopson

Dr. Vopson is Senior Lecturer in Physics, in the School of Mathematics and Physics at the University of Portsmouth, UK. In his current role he developed the Applied Materials Research Laboratory and various academic teaching modules including a unique university course on Multiferroic Materials and Their Applications.
His previous appointments include two postdoctoral fellowships at the University of York, senior R&D scientist at Seagate Technology and over six years Higher Research Scientist at the UK National Physical Laboratory.
Dr. Vopson published over 90 research articles, he has an h-index of 21 and he received over 1900 citations [https://scholar.google.co.uk/citations?user=09NGMwcAAAAJ&hl=en]. His major scientific contributions are in the fields of solid state caloric effects, thin film growth technologies, multiferroic materials and their applications, optical techniques of characterization of solids, development of novel metrologies and innovations based on ferroic materials, theoretical studies of non-equilibrium phenomena, fundamental physics and information physics.
Disclaimer: The views expressed here are those of the interviewee and do not necessarily represent the views of AZoM.com Limited (T/A) AZoNetwork, the owner and operator of this website. This disclaimer forms part of the Terms and Conditions of use of this website.