A majority of quantum information tools, including quantum computers, which are regarded as a level above supercomputers, and quantum communication that is unable to be hacked are founded on the quantum entanglement principle. Nevertheless, entangled systems are present in a small microscopic world, and they are quite delicate.
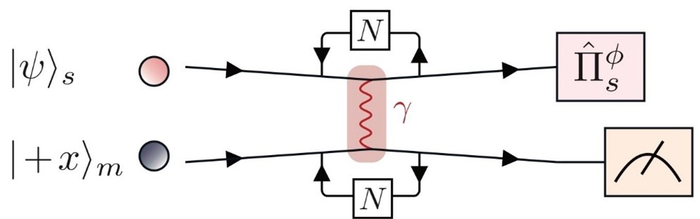A schematic of iterative interaction between the quantum system to be measured and the quantum system of the measuring device. Image Credit: Pohang University of Science and Technology.
Quantum metrology, providing improved sensitivity over traditional measurements in precision metrology, has also primarily depended on quantum entanglement, so it is difficult to put it into practice in real-time applications. Recently, a Korean research group has suggested an approach to gain quantum metrology accuracy without employing entangled resources.
A POSTECH research group headed by Professor Yoon-Ho Kim and Dr. Yosep Kim (Department of Physics) has found a weak-value amplification (WVA) approach that arrives at the Heisenberg limit without employing quantum entanglement. Heisenberg-limit refers to the accuracy that is finally attainable in quantum metrology.
WVA-based metrology is one of the approaches for quantifying quantum effects. This metrology is a method to gain the most information as possible on the quantum system with minimum effect. It can measure the system efficiently without damaging the quantum state.
Using the weak value measured in this way, it is possible to amplify small physical impacts, like ultrasmall phase shifts. This approach has a smaller amount of errors in comparison to the traditional methods; however, it has a serious limitation of lesser detection possibility.
Utilizing entanglement proposes approaches to overcome this drawback, but the challenge in producing a large-scale quantum entanglement has been a big issue when attempting to realize the Heisenberg-limited metrology.
The team has assured that the Heisenberg limit is reached in the weak-value amplification, without employing entanglement by the iterative interaction among different quantum states. It explains that this is due to the local iterative interactions among every particle of an entangled system and a meter, and not from the quantum entanglement itself.
Professor Yoon-Ho Kim headed the study.
This study will contribute to the practical use of quantum metrology by verifying that entanglement is not an absolute requirement for reaching the Heisenberg limit.
Professor Yoon-Ho Kim, Department of Physics, Pohang University of Science and Technology
Journal Reference:
Kim, Y., et al., (2022) Heisenberg-Limited Metrology via Weak-Value Amplification without Using Entangled Resources. Physical Review Letters. doi.org/10.1103/PhysRevLett.128.040503.