Jan 19 2016
Knot solitons, or knotted solitary waves, have been successfully created in a quantum-mechanical field that describes a gas of superfluid atoms, known as Bose-Einstein condensate.
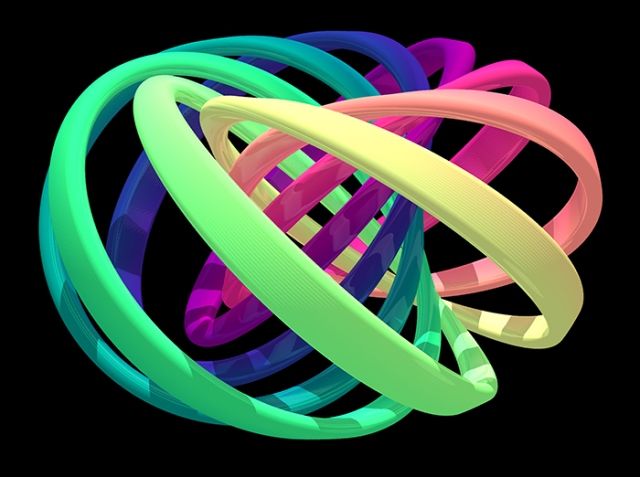Visualization of the structure of the created quantum knot. Each colorful band represents a set of nearby directions of the quantum field that is knotted. Note that each band is twisted and linked with the others once. Untying the knot requires the bands to separate, which is not possible without breaking them. See instruction below to access the full-resolution image. Credit: David Hall.
The results of the study, conducted by researchers from Aalto University and Amherst College, have appeared in the journal, Nature Physics.
The quantum knots exist in a field, which assume a specific direction in every space point as opposed to knotted ropes. The field separates into an infinate number of rings, and every ring has its own field direction. This yields a topologically stable structure, as the rings need to be broken in order to separate this structure. The knots present within the superfluid can only be untied if the state of the quantum matter is destroyed.
To make this discovery we exposed a Rubidium condensate to rapid changes of a specifically tailored magnetic field, tying the knot in less than a thousandth of a second. After we learned how to tie the first quantum knot, we have become rather good at it. Thus far, we have tied several hundred such knots.
Professor David Hall, Amherst College
To tie the knot, the structure was squeezed into the condensate from its boundaries. The quantum field needed to be initialized in a specific direction to acheive this, and followed by a sudden change in the applied magnetic field in order to establish an isolated null point, where the magnetic field disappears into the cloud center. The knot was then tied by the magnetic field within a millisecond.
For decades, physicists have been theoretically predicting that it should be possible to have knots in quantum fields, but nobody else has been able to make one. Now that we have seen these exotic beasts, we are really excited to study their peculiar properties. Importantly, our discovery connects to a diverse set of research fields including cosmology, fusion power, and quantum computers.
Mikko Möttönen, Aalto University
Knots have been used and recognized by people for thousands of years. A source of inspiration for many complex patterns and designs, in addition to enabling great seafaring endeavors. A system of knots, called quipu, was used by the Inca civilization in the ancient times for information storage. More recently, the role of knots has been considered to be important in the quantum-mechanical fundamentals of nature. They have remained unseen in the field of quantum dynamics.
The knots that we come across in our daily life are tied on strings or ropes with two ends. It is possible to untie such knots without cutting the strings, so they are defined as not topologically stable by mathematicians. The rope ends are glued together to form stable knots and it is not possible to untie such knots without using scissors. However, relocation of the knots within the rope is possible.
To describe a quantum knot mathematically, a mapping known as Hopf fibration is realized by the created quantum knot. The Hopf fibration, defined by Heinz Hopf in 1931, remains the subject of intense research in the fields of physics and mathematics. For the first time the researchers were able to experimentally demonstrate this phenomenon in a quantum field.
This is the beginning of the story of quantum knots. It would be great to see even more sophisticated quantum knots to appear such as those with knotted cores. Also it would be important to create these knots in conditions where the state of the quantum matter would be inherently stable. Such system would allow for detailed studies of the stability of the knot itself.
Mikko Möttönen, Aalto University