In an article recently published in the journal Scientific Reports, researchers used novel techniques to investigate the dynamic behavior of new types of nonlinear fractional dynamical systems with chaotic parameters and chaos-coherence exponents. The objective was to study the partially chaos hybrid systems to simulate the nonlinear phenomena’s experimental applications.
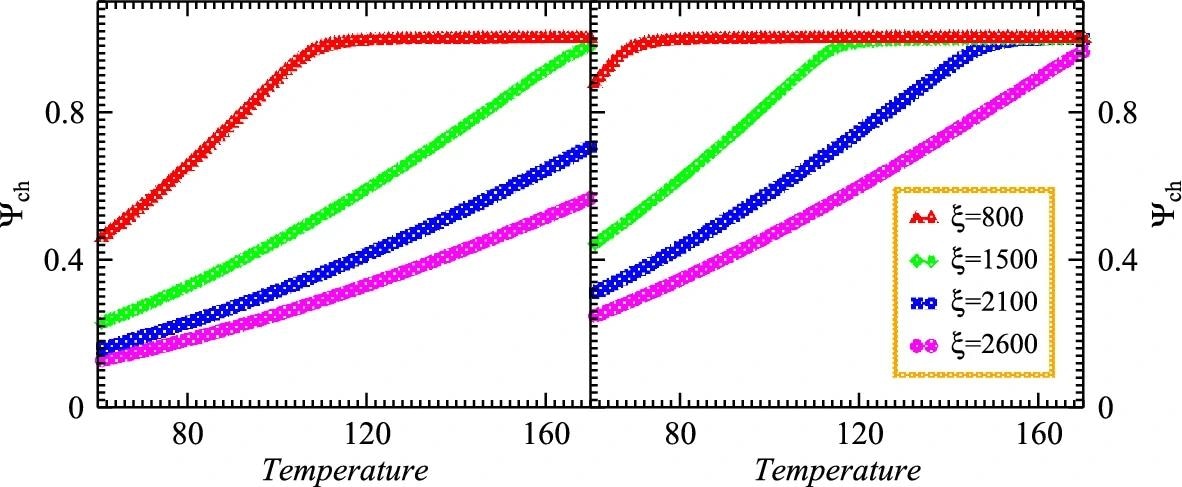Chaotic emission spectra versus temperatures for the nonlinear systems with different source sizes and multiplicities. Image Credit: https://www.nature.com/articles/s41598-024-61588-0
Quantum Chaos and Nonlinear Dynamics
Interferometry proves to be an effective tool for evaluating the complex evolution of dynamical systems associated with long-term memory effects and for analyzing the structure and phase transitions of physical systems in low temperature and low momentum regimes, a topic of interest in nonlinear dynamics.
Significant transitions manifest through quantum chaos-coherence at specific temperatures, with the effects of these transitions dependent on unique elements such as the condensation fraction degree, chaos, multiplicities, and spin. The normalized chaotic-coherent parameters are introduced to illustrate the nonlinear phenomena at zero relative momenta within the quantum interferences among multi-emitted identical particles generated within the nonlinear systems.
These correlations appear in chaotic phenomena and vanish in coherent emissions, exploring the systems' peculiarities. Thus, the results of the coherent-chaotic parameters correlate with the systems' chaos-coherent degree. The specific relationship between the environment and parameters for the considered nonlinear systems facilitates a deeper understanding of the interferometry data.
Furthermore, the concept of quantum chaos-coherence, arising from superpositions, is essential for understanding the divergent correlations and quantum interferences in physical systems. Various methods have been investigated to evaluate chaos at different temperatures and momentum regimes. These efforts revealed that dynamic relations between chaotic parameters and quantum coherence exhibit inherent peculiarities concerning nonlinear systems.
Recently, there has been a surge of interest in studying the dynamics of systems with fractional derivatives, focusing on their stability, complexity, chaotic behavior, and synchronization. The findings facilitate the examination of parameters and the clarification of complex factors to better understand the system dimensions.
The Study
This study examined novel approaches within multi-scale properties and coherent-chaotic domains for nonlinear phenomena. Researchers investigated different higher-order chaotic parameters to gain deeper insights into the inherent properties of nonlinear systems.
The system peculiarities were examined through chaotic parameters, condensation, and chaotic parameters. Additionally, the source-dependent and coherent captured particle emissions were analyzed to understand the pattern formation.
Researchers demonstrated normalized chaos parameters and momentum-dependent density distributions along with the systems' structure analysis. Several familiar chaotic systems were extended through the implementation of generalized higher-order methodologies. The propagation of particularism with ample energy to travel from generation to observation points was examined.
Exploring the nonlinear systems in nonlinear phenomena was necessary using interferometric techniques. Researchers characterized the studied systems as chaotic when the level of coherence appeared negligible. This method revealed the propensity for chaos at various relative momenta. Consequently, there was a growing interest in investigating partially chaotic systems due to their real-world implications and prevalence in various fields like biology, engineering, and physics.
The dynamic behavior of new types of nonlinear fractional dynamical systems with chaos-coherence exponents and chaotic parameters was explored. Specifically, the collective dynamics of these systems were analyzed in the presence of partial chaotic peculiarities. Correlations, along with their corresponding coherent-chaotic parameters, were computed.
Such parameters have clear relationships with the condensates. This allowed researchers to characterize the systems and investigate the influence of temperature, chaos, momentum, and multiplicities of the system on the coherent-chaotic normalized correlations.
Thus, nonlinear dynamical analysis serves as a tool to unveil the quantum-mechanical peculiarities, probing the systems' intrinsic characteristics. Hence, complex complications within these systems can be interpreted through nonlinear dynamics. Researchers modeled the new chaotic systems and proposed efficient techniques for numerically simulating the investigated fractional systems, which included both chaos and coherence fractions.
Significance of the Study
The chaotic parameters were considerably suppressed with the coherence fraction, with the chaotic parameter appearing numerically zero at maximum condensation and one at ideal chaos emissions. Meaningful parameters were reduced significantly with the multiplicity of the nonlinear systems and increased with momentum in the specified regimes.
The presence of identical multiplicities prompted the researchers to consider the role of coherence. Consequently, the significant influence these identical multiplicities have on the normalized chaotic parameters warranted further investigation. The techniques remained categorical, dependable, and intelligible to correlate in different quantum phenomena.
Additionally, the key characteristics of the nonlinear system's peculiarities and the consequences were extremely consistent with the droplets and ascendance of the temperature regimes, indicating potential applications in engineering and medical fields. Overall, the findings highlighted the importance of analyzing correlations to decipher the characteristics of nonlinear systems. By doing so, we gain remarkable insight into the intricate nature of complex systems.
Journal Reference
Bary, G., Ahmed, W., Ahmad, R., Niazai, S., Khan, I. (2024). Novel techniques to analyze dynamical properties of quantum chaos with peculiar evidence of hybrid systems confinement. Scientific Reports, 14(1), 1-18. https://doi.org/10.1038/s41598-024-61588-0, https://www.nature.com/articles/s41598-024-61588-0
Disclaimer: The views expressed here are those of the author expressed in their private capacity and do not necessarily represent the views of AZoM.com Limited T/A AZoNetwork the owner and operator of this website. This disclaimer forms part of the Terms and conditions of use of this website.