Jan 28 2016
Quantum objects cannot be understood simply as the sum of their parts, making quantum calculations complicated. A single hydrogen atom can easily be calculated, but rough approximations are often required to define an atom cloud of several thousands of atoms, as it is not possible to describe the quantum particles separately due to their interconnection with each other.
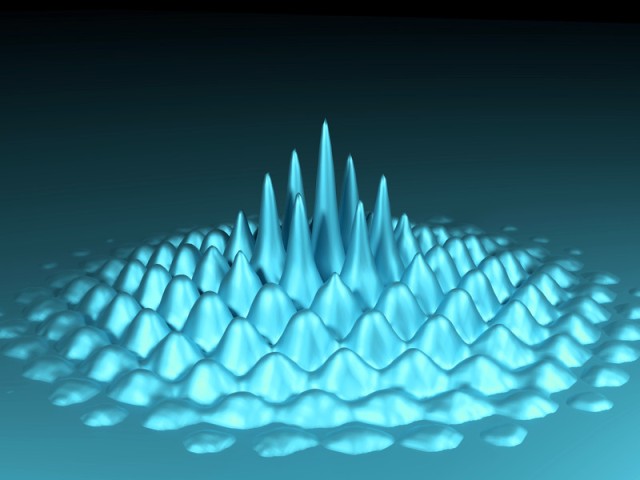Bose-Einstein-condensates making waves: a many-particle phenomenon (credit: TU Wien)
To overcome this problem, Mark Kasevich from Stanford, USA, and Kaspar Sakmann from TU Wien, Vienna, have proposed a solution in a paper reported in the journal, Nature Physics. They have successfully calculated the effects in the ultra-cold atom clouds, which can only be described in terms of the quantum correlations existing between many atoms.
Quantum Correlations
Quantum physics is a game of randomness and luck. Like the resulting number on a die whirling in the air, the positions of atoms in the cold atom cloud are not predefined. In the cold atom cloud, the atoms occupy multiple locations at the same time. The locations are fixed only when measuring the atoms.
We shine light on the atom cloud, which is then absorbed by the atoms. The atoms are photographed, and this is what determines their position. The result is completely random.
Kaspar Sakmann, TU Wien, Vienna
A die game is different to quantum randomness, when two dice are rolled together in the air simultaneously they can appear as two independent dice to the user. The result of one die is not affected by the other die. The atoms present in the cold atom cloud are actually a big quantum object as they are quantum physically connected, therefore it is meaningless to study the atoms separately.
Hence, the measurement of a single atom's position is related in a mathematically complicated manner to the positions of other existing atoms.
It is not hard to determine the probability that a particle will be found at a specific position. The probability is highest in the centre of the cloud and gradually diminishes towards the outer fringes.
Kaspar Sakmann, TU Wien, Vienna
We know that when we throw a die, the result could be any number with a probability of one sixth. The probability of getting three ones when rolling three dice can also be determined, and the probability will remain the same if we roll five ones repeatedly. However, calculations are more complex in the case of quantum particles.
We solve this problem step by step. First we calculate the probability of the first particle being measured on a certain position. The probability distribution of the second particle depends on where the first particle has been found. The position of the third particle depends on the first two, and so on.
Kaspar Sakmann, TU Wien, Vienna
The last quantum particle's location can be defined when the positions of all other particles are known. As a result of this kind of quantum entanglement, mathematically calculating the positions of quantum particles becomes very difficult.
Only Correlations Can Explain the Experimental Data
The relationship between the quantum particles is more important for estimating the colloidal behavior of Bose-Einstein condensates.
The experiment shows that such collisions can lead to a special kind of quantum waves. On certain positions we find many particles, on an adjacent position we do not find any. If we consider the atoms separately, this cannot be explained. Only if we take the full quantum distribution into account, with all its higher correlations, these waves can be reproduced by our calculations.
Kaspar Sakmann, TU Wien, Vienna
The same procedure is used to calculate other phenomena such as the Bose-Einstein-condensates, which show the emergence of little vortices, a typical quantum many-particle-effect, upon stirring with a laser beam.
Our results show how important theses correlations are and that it is possible to include them in quantum calculations, in spite of all mathematical difficulties.
Kaspar Sakmann, TU Wien, Vienna
According to the researchers, it is possible to apply this method on other quantum systems as well after certain modifications.
Source: http://www.tuwien.ac.at/